历史

定义

平面曲线

在数学上，一条曲线的定义为：

我们常遇到的平面曲线的拓扑空间为 R 2 {\displaystyle \mathbb {R} ^{2}} 。

空间曲线

常见曲线

请参见曲线列表

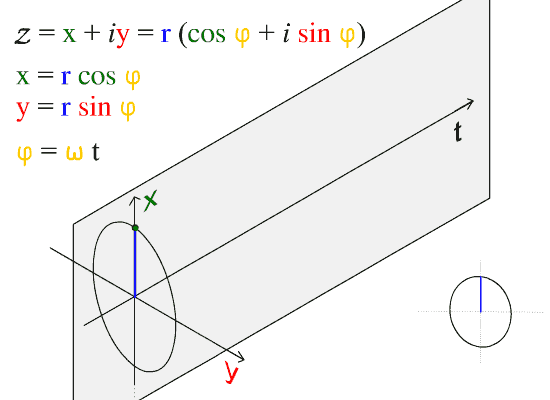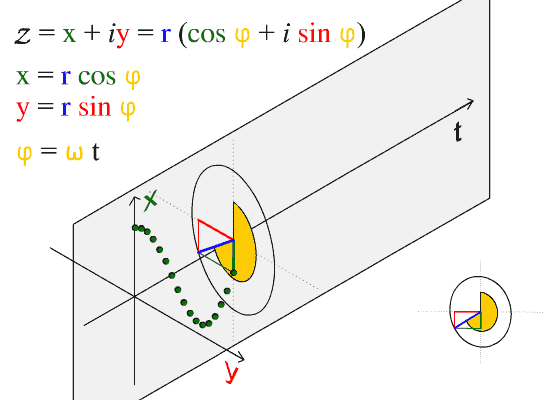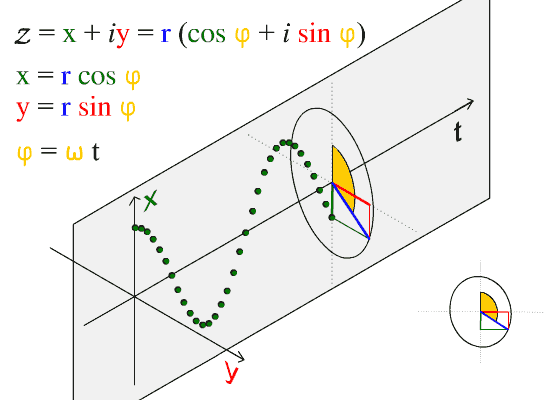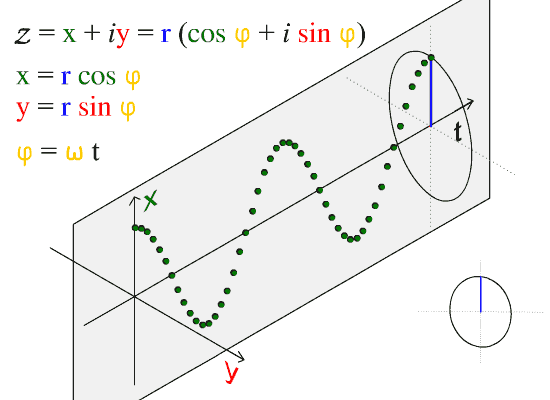
曲线方程

请参阅参数方程。

一般来说，当在 R n {\displaystyle \mathbb {R} ^{n}} 下一些符合一条方程的点的集合组成一条曲线时，那方程就叫那曲线的 曲线方程 。

例如， x 2 + y 2 = 1 {\displaystyle x^{2}+y^{2}=1} 是单位圆的曲线方程，因为有且仅有单位圆上的点符合这条方程；因这些点组成一个单位圆，故该方程正代表着平面上的单位圆。

曲线的长度

平面曲线

若一条平面曲线可表达成标准方程 y = f ( x ) {\displaystyle y=f(x)\,} ，那么它的长度就是：

其中 a {\displaystyle a\,} 、 b {\displaystyle b\,} 为 x {\displaystyle x\,} 的上下限。

若平面曲线可表达成参数方程 { x = x ( t ) y = y ( t ) {\displaystyle \left\{{\begin{matrix}x=x\left(t\right)\\y=y\left(t\right)\end{matrix}}\right.} 。，那么它的长度就是：

其中 α α --> {\displaystyle \alpha \,} 、 β β --> {\displaystyle \beta \,} 为 t {\displaystyle t\,} 的上下限。

空间曲线

免责声明：以上内容版权归原作者所有，如有侵犯您的原创版权请告知，我们将尽快删除相关内容。感谢每一位辛勤著写的作者，感谢每一位的分享。